Galxe login | Galxe ID | Passport | Explore Campaigns | Galxe products
Galxe login | Galxe ID | Passport | Explore Campaigns | Galxe products
Galaxy polyhedra are remarkable celestial structures that have captivated the imagination of scientists and stargazers alike. These intricate formations combine the beauty of galaxies with the geometric elegance of polyhedra, creating visually stunning and mathematically intriguing objects. In this article, we will delve into the realm of galaxy polyhedra, exploring their origin, formation, and the fascinating properties that make them unique.
Galaxy polyhedra, also known as polyhedral galaxies, are a special class of galaxies that exhibit a strikingly symmetric and polyhedral shape. Unlike the more common spiral or elliptical galaxies, these celestial objects feature well-defined geometric shapes such as cubes, dodecahedra, and icosahedra. The intricate network of stars, gas, and dust within these polyhedral galaxies is arranged in a way that mimics the faces, vertices, and edges of these geometric solids.
The formation of galaxy polyhedra is still a subject of ongoing research and debate among astrophysicists. One hypothesis suggests that these structures arise from a complex interplay of gravitational forces, mergers between galaxies, and subsequent interactions with neighboring objects. These processes can lead to the rearrangement of stars and gas into the distinctive polyhedral shapes that we observe today.
One of the most intriguing aspects of galaxy polyhedra is their striking visual appearance. The symmetrical nature of these celestial objects is visually captivating, with their sharp edges and well-defined faces contrasting against the vast cosmic backdrop. The interaction between light and matter within these polyhedra can give rise to stunning color patterns and light reflections, further enhancing their beauty and allure.
Galxe polyhedra, also known as spherical polyhedra, are a fascinating geometric concept that has captured the attention of mathematicians and artists throughout history. These intricate structures are composed of a collection of polygons that fit together perfectly to form a closed surface on the sphere.
The origins of galxe polyhedra can be traced back to ancient Greece, where mathematicians like Plato and Archimedes explored the symmetries and properties of regular polyhedra. However, it wasn't until the 19th century that the study of spherical polyhedra gained significant attention.
During this time, mathematicians such as Julius Petersen and Karl Reinhardt began to delve into the world of spherical polyhedra, publishing groundbreaking works that laid the foundation for future research in the field. These early pioneers not only explored the mathematical properties of galxe polyhedra but also delved into their aesthetic appeal.
One of the most famous examples of galxe polyhedra can be found in M.C. Escher's artwork. Escher was widely known for his mind-bending optical illusions and intricate tessellations, many of which featured spherical polyhedra. His work brought galxe polyhedra into the mainstream and sparked further interest in their geometric properties.
Today, the study of galxe polyhedra continues to be an active area of research in mathematics and computer science. Mathematicians are constantly discovering new types of spherical polyhedra and studying their symmetries and characteristics. These structures have applications in various fields, including architecture, biology, and even chemistry.
In conclusion, the history and origins of galxe polyhedra are deeply rooted in ancient Greece but have evolved over time thanks to the contributions of mathematicians, artists, and researchers. Their exploration has not only expanded our understanding of geometry but has also influenced various artistic and scientific fields.
Galxe polyhedra are a fascinating class of geometric shapes that possess unique and mesmerizing properties. These three-dimensional structures, also known as duals of polyhedral compounds, are formed by interlocking regular polygons and exhibiting truly captivating patterns.
One of the most intriguing features of galxe polyhedra is their symmetrical nature. Each face of these polyhedra is identical, resulting in a balanced and harmonious structure. This symmetrical property not only adds to their aesthetic appeal but also gives them a sense of stability and equilibrium.
Furthermore, galxe polyhedra have a remarkable ability to transform and morph into different shapes. By adjusting the angles and positions of their constituent polygons, these structures can change their forms and create an astonishing variety of intricate patterns. This flexibility and adaptability make galxe polyhedra a playground for mathematical exploration and artistic expression.
In addition to their visual allure, galxe polyhedra also have practical applications in various fields. Their unique geometries can be found in architectural designs, molecular structures, and even in the study of crystallography. Understanding the properties and behaviors of galxe polyhedra can provide valuable insights into the nature of shapes and their interactions.
Exploring the fascinating world of galxe polyhedra opens up a whole new realm of imagination and discovery. These structures not only captivate the eye with their mesmerizing patterns but also challenge our understanding of geometry and its possibilities. Whether in art, science, or mathematics, galxe polyhedra continue to inspire and intrigue, revealing the endless possibilities of shapes and their hidden beauty.
Galxe polyhedra are a fascinating class of geometric structures that have captured the attention of scientists and mathematicians alike. With their intricate network of edges and vertices, galxe polyhedra offer a multitude of unique structural characteristics that make them worthy subjects of study.
One of the defining features of galxe polyhedra is their highly symmetrical arrangement. Each face of a galxe polyhedron is identical in shape and size, resulting in a uniform appearance. This symmetry gives galxe polyhedra a mesmerizing aesthetic appeal, making them visually stunning objects to explore.
Another intriguing aspect of galxe polyhedra is their exceptional stability. Due to their symmetrical structure, galxe polyhedra have a balanced distribution of forces, making them resistant to collapsing or deforming under external pressures. This stability makes galxe polyhedra suitable for a variety of applications, such as architectural design and material engineering.
Furthermore, galxe polyhedra exhibit a remarkable level of connectivity among their vertices and edges. Each vertex in a galxe polyhedron is connected to a specific number of edges, creating a complex web of interconnections. This connectivity is essential for understanding the flow and distribution of energy, information, or other resources within a galxe polyhedron.
To better visualize and explore the structural characteristics of galxe polyhedra, researchers and enthusiasts can utilize advanced computational tools and simulations. These tools provide a comprehensive understanding of the intricate internal arrangements of galxe polyhedra, allowing for further analysis and experimentation.
In conclusion, galxe polyhedra offer a captivating glimpse into the world of geometry and mathematics. Their unique structural characteristics, including symmetry, stability, and connectivity, make them a fascinating subject of study. If you're curious to learn more about galxe polyhedra and their applications, check out What does Galxe do? for more information.
Galxe polyhedra, with their unique geometric structures and properties, have found numerous applications in various fields of modern science and technology. Their intricate design and symmetrical arrangements make them ideal for a wide range of applications, including:
Materials Science and Engineering: Galxe polyhedra can be used as templates to create new materials with tailored properties. By controlling the size, shape, and surface chemistry of the polyhedra, scientists can create materials with enhanced strength, conductivity, or catalytic activity. These materials find applications in areas such as aerospace, electronics, and renewable energy.
Nanotechnology: Galxe polyhedra serve as building blocks for creating nanostructures with precise control over their size and shape. They can be assembled into lattices or used as scaffolds for growing nanowires or nanoparticles. These nanostructures have potential applications in electronics, photonics, and biotechnology.
Drug Delivery Systems: The unique properties of galxe polyhedra, such as their large surface area and porosity, make them suitable for drug delivery systems. Drugs can be loaded onto the surface or within the cavities of the polyhedra and released in a controlled manner. This offers advantages such as targeted drug delivery, improved drug stability, and reduced side effects.
Catalysis: Galxe polyhedra can be functionalized with different catalysts to enhance their catalytic activity. They can act as efficient catalysts for various chemical reactions, such as hydrogenation, oxidation, and polymerization. This has implications in industrial processes, environmental remediation, and renewable energy production.
Data Storage: The unique symmetry and arrangement of galxe polyhedra can be used for data storage applications. By encoding information into the geometry or arrangement of polyhedra, researchers are exploring the possibility of creating high-density storage devices with increased capacity and stability.
These are just a few examples of the applications of galxe polyhedra in modern science and technology. With further research and development, it is expected that their utilization will continue to expand, unlocking new possibilities and advancements in various fields.
Galxe polyhedra have played a significant role in mathematical research and discovery. These unique structures have captured the attention of mathematicians for centuries, offering a rich playground for exploring geometric properties and mathematical principles.
One of the key contributions of galxe polyhedra to mathematical research is their ability to expand our understanding of geometries and dimensions. These complex shapes, often consisting of interconnected polygons, challenge traditional Euclidean geometry and offer new perspectives on space and shape. By examining the relationships between the faces, edges, and vertices of galxe polyhedra, mathematicians have been able to uncover new insights into the nature of geometry itself.
Galxe polyhedra also provide a fertile ground for discovering hidden symmetries and patterns. By studying the symmetries present in these intricate structures, mathematicians have been able to uncover regular and irregular patterns that were previously unknown. These symmetries often have deep connections to other areas of mathematics, such as group theory and topology, and can reveal unexpected connections between different branches of mathematical research.
Moreover, galxe polyhedra have proven to be a valuable tool for investigating the nature of space and its transformations. By examining how these polyhedra can be transformed through rotations, translations, and reflections, mathematicians can gain valuable insights into the fundamental principles that govern spatial transformations.
Exploration of complex geometries
Truncated icosahedron, rhombicosidodecahedron
Identification of hidden symmetries
Truncated tetrahedron, snub dodecahedron
Analysis of spatial transformations
Cuboctahedron, great icosahedron
In conclusion, galxe polyhedra have become invaluable tools in the world of mathematical research and discovery. Their unique properties and intricate structures offer mathematicians new avenues for exploration and help deepen our understanding of geometry, symmetry, and spatial transformations.
Galxe polyhedra exhibit a fascinating array of shapes and forms, which make them a captivating subject of study within the realm of geometry. These three-dimensional structures, composed of interconnected polygons, have captured the attention of mathematicians, physicists, and artists alike.
One notable characteristic of galxe polyhedra is their ability to possess multiple faces, vertices, and edges. These features contribute to their complexity and diversity, leading to a wide range of possible configurations.
Upon closer analysis, it becomes clear that galxe polyhedra can be classified into various categories based on their shape and structural properties. Some polyhedra exhibit symmetrical patterns, while others display irregular or asymmetrical arrangements.
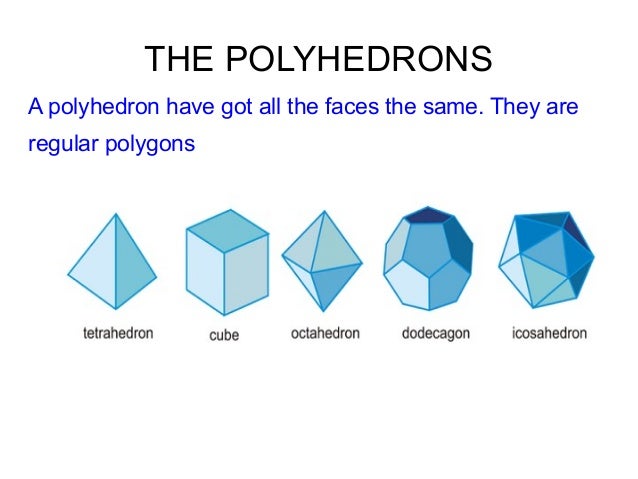
The Platonic solids, such as the tetrahedron, octahedron, and dodecahedron, are examples of symmetrical galxe polyhedra that possess equal faces, vertices, and edges. Their geometric perfection has made them highly regarded in mathematics and aesthetics.
In contrast, the Archimedean solids, including the truncated icosahedron and rhombicuboctahedron, exhibit a greater degree of complexity. These polyhedra have different types of faces, resulting in a more varied combination of shapes.
The versatility of galxe polyhedra is further demonstrated through their transformational properties. By truncating, stellating, or chamfering the edges or faces of a given polyhedron, new forms can be created. These transformations not only enhance the aesthetic appeal of the polyhedra but also provide insight into the underlying mathematical principles governing their construction.
To fully understand the diverse shapes and forms of galxe polyhedra, it is essential to delve into their mathematical properties. The study of vertex counts, face arrangement, and edge lengths enables mathematicians to classify and analyze these intricate structures.
By representing galxe polyhedra using graphs or matrices, researchers can explore their connectivity and symmetry. This approach allows for a more systematic analysis, providing a deeper understanding of the underlying geometric relationships within the polyhedra.
The captivating shapes and forms of galxe polyhedra are not limited to the realm of mathematics. These structures can be found in various forms of natural phenomena, such as crystal structures and biological organisms.
Additionally, artists have long been inspired by the mesmerizing beauty of galxe polyhedra. From architectural designs to sculptures and paintings, the intricate geometries of these polyhedra have been incorporated into artistic creations throughout history.
Tetrahedron
A polyhedron with four triangular faces.
Octahedron
A polyhedron with eight triangular faces.
Dodecahedron
A polyhedron with twelve pentagonal faces.
Truncated icosahedron
A polyhedron with twenty hexagonal and twelve pentagonal faces.
Rhombicuboctahedron
A polyhedron with eighteen square and eight triangular faces.
Through the analysis of the diverse shapes and forms of galxe polyhedra, we gain a deeper appreciation for their complexity and beauty. These structures continue to intrigue and inspire further research, offering new insights into the fascinating realm of geometry.
Galxe polyhedra are intricate and complex geometric shapes that have captivated the minds of mathematicians and scientists for centuries. These polyhedra, often referred to as "spacefilling polyhedra," have the ability to fill three-dimensional space without leaving any gaps or overlaps.
One intriguing aspect of galxe polyhedra is their connection to fractals. Fractals are mathematical patterns that repeat themselves at different scales, creating intricate and self-similar structures.
When we examine galxe polyhedra closely, we can observe fractal-like properties within their structure. The intricate patterns and self-similarity found in galxe polyhedra can be seen at various levels of magnification, just like fractals.
This connection between galxe polyhedra and fractals opens up a fascinating realm of exploration and study. Mathematicians and scientists are now delving deeper into this connection, uncovering the underlying principles and mathematical equations that govern both galxe polyhedra and fractals.
The study of galxe polyhedra and fractals requires a deep understanding of complex mathematical concepts. Mathematicians use advanced algebra, geometry, and calculus to analyze and describe the intricate patterns and structures found within galxe polyhedra and fractals.
One area of focus is the concept of self-similarity. Fractals exhibit self-similarity, where smaller parts of the structure resemble the whole structure. Galxe polyhedra share this property, with smaller components within the polyhedra mirroring the overall shape and structure.
The connection between galxe polyhedra and fractals has numerous implications and applications in various fields. It can have implications in architecture and design, as the complex and visually appealing patterns of galxe polyhedra and fractals can inspire unique and innovative structures.
Furthermore, understanding the mathematics behind galxe polyhedra and fractals can have applications in computer graphics and visualization. By incorporating fractal-like properties and galxe polyhedra structures, computer-generated images and simulations can achieve a higher level of realism and complexity.
In conclusion, the connection between galxe polyhedra and fractals is truly fascinating. These complex geometric shapes share fractal-like properties, allowing for further exploration and understanding of both subjects. This connection has implications in various fields, highlighting the importance of studying and researching galxe polyhedra and fractals.
The realm of galxe polyhedra holds immense potential for future developments and advancements in various fields. As researchers continue to delve deeper into the intricacies of these fascinating structures, new applications and innovative approaches are being explored.
One of the key areas where galxe polyhedra is expected to have a significant impact is in materials science. These unique geometrical patterns hold promise for the creation of new materials with unprecedented properties. By manipulating the arrangement of atoms and molecules within galxe polyhedra, it is possible to design materials with exceptional strength, conductivity, and other desirable characteristics.
Furthermore, the study of galxe polyhedra opens avenues for advancements in the field of drug delivery systems. The complex architectures of these structures can be utilized to encapsulate drugs and release them in a controlled manner within the body. This can potentially revolutionize the field of medicine, allowing for targeted, efficient, and precise drug delivery, minimizing side effects and maximizing therapeutic efficacy.
Additionally, the understanding of galxe polyhedra has the potential to inform advancements in the field of nanotechnology. These intricate structures could serve as building blocks for the creation of nanoscale devices and systems. By harnessing the unique properties of galxe polyhedra, such as their stability and self-assembling capabilities, researchers can pave the way for the development of miniaturized technologies with applications ranging from electronics to biomedical engineering.
In the future, the study of galxe polyhedra can also lead to breakthroughs in our understanding of fundamental scientific principles. The exploration of their mathematical properties and symmetries can shed light on the underlying principles of geometry and topology. This knowledge can have implications in various fields, including physics, mathematics, and engineering, fostering interdisciplinary collaborations and pushing the boundaries of scientific knowledge.
In conclusion, galxe polyhedra hold immense promise for the future. With continued research and exploration, these captivating structures have the potential to revolutionize materials science, drug delivery systems, nanotechnology, and our fundamental understanding of science. The future prospects in this field are both exciting and limitless.
What are galxe polyhedra?
Galxe polyhedra are geometric structures composed of polygons, typically triangles, that are connected at their vertices to form a three-dimensional shape.
How are galxe polyhedra different from other polyhedra?
Galxe polyhedra differ from other polyhedra in that they have a more complex and intricate structure, often with irregular polygonal faces and a higher number of vertices.
What are some common applications of galxe polyhedra?
Galxe polyhedra have various applications in fields such as architecture, sculpture, and computer graphics. They are often used to create visually stunning and unique designs.
Can galxe polyhedra be found in nature?
While galxe polyhedra are primarily man-made structures, there are some natural formations that can resemble these geometric shapes, such as certain crystal formations and the structure of some viruses.
How can one create their own galxe polyhedra?
Creating galxe polyhedra can be a complex process, but it typically involves assembling individual polygons, such as triangles, into a three-dimensional structure. Various software programs and 3D modeling techniques can also be used to design and create galxe polyhedra.
Galxe login | Galxe ID | Passport | Explore Campaigns | Galxe products
2022-2024 @ An in depth look into the captivating realm of galxe polyhedra
