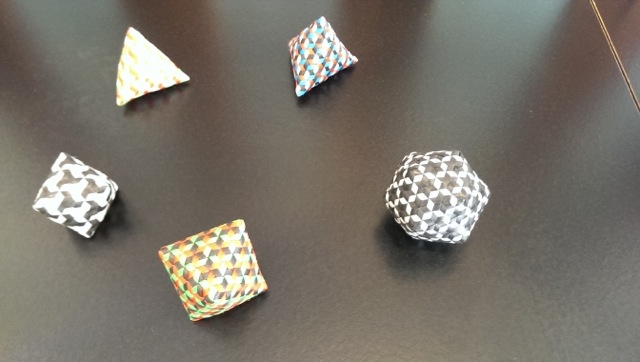
Galxe polyhedra are fascinating mathematical objects that have been studied for centuries. These three-dimensional shapes, also known as star polyhedra, are formed by connecting the vertices of a regular polyhedron with straight lines. The resulting structure is a network of triangles and pentagrams that create intricate patterns and symmetries.
The study of galxe polyhedra is deeply rooted in the field of geometry. Mathematicians have long been interested in understanding the properties and characteristics of these complex shapes. Through rigorous proofs and mathematical reasoning, they have been able to unravel the mysteries behind galxe polyhedra and shed light on their fascinating nature.
One of the central concepts in the study of galxe polyhedra is symmetry. These shapes exhibit a wide range of symmetries, from rotational symmetry to mirror symmetry. By analyzing the symmetries of galxe polyhedra, mathematicians have been able to classify and categorize them into different families and types.
The theories and proofs behind galxe polyhedra have practical applications in various fields, including architecture, crystallography, and graphic design. Understanding the mathematical principles that govern these shapes can help designers create aesthetically pleasing and structurally sound structures. Furthermore, the study of galxe polyhedra has also led to advancements in computational geometry, providing algorithms and methods for manipulating and analyzing these complex objects.
Polyhedra have been studied and admired since ancient times. The origins of geometric polyhedra can be traced back to the early civilizations of Egypt, Mesopotamia, and Greece. These civilizations had a deep fascination with geometry and its applications in architecture, art, and mathematics.
One of the earliest known examples of polyhedra is the Egyptian pyramid. The pyramids were constructed as tombs for pharaohs, and their design and construction required a deep understanding of geometry. The pyramids are examples of regular polyhedra, also known as Platonic solids. These polyhedra have unique properties, such as regularity of angles and faces, and have fascinated mathematicians for centuries.
In ancient Mesopotamia, the Babylonians were also fascinated by geometry. They used geometric shapes such as triangles, squares, and rectangles in their architecture and art. They also developed mathematical methods for calculating the area and volume of these shapes, laying the foundations for the development of modern geometric principles.
The ancient Greeks took the study of geometry to new heights. Figures such as Pythagoras, Euclid, and Archimedes made significant contributions to the understanding of geometric polyhedra. Euclid's book "Elements" is considered one of the most important works in the history of mathematics, as it laid down the foundations of geometry as a formal mathematical discipline. Archimedes, on the other hand, made discoveries about the volume and surface area of various polyhedra, including the sphere and the cone.
One of the key aspects of studying geometric polyhedra is understanding their symmetry. Symmetry refers to the balance and proportion in a shape or object. The ancient Greeks were particularly interested in symmetry and its mathematical properties. They developed the concept of symmetry groups, which describe the different ways in which a shape can be transformed while preserving its overall structure.
Modern mathematics has built upon the foundation laid by these ancient civilizations. The study of geometric polyhedra has expanded to include complex structures such as fractals and non-Euclidean geometries. Today, geometric polyhedra continue to captivate mathematicians, artists, and scientists alike, as they represent the timeless beauty and elegance of mathematical principles.
The origins of geometric polyhedra can be traced back to the ancient civilizations of Egypt, Mesopotamia, and Greece. These cultures had a deep appreciation for geometry and its applications in various fields. The study of geometric polyhedra has evolved over time and continues to be a fascinating area of research in modern mathematics. Understanding the origins of geometric polyhedra helps us appreciate the richness and beauty of mathematical principles.
Polyhedra, also known as three-dimensional geometric figures, have fascinated mathematicians and scientists for centuries. In the field of mathematics, polyhedra are studied and explored through various theories and proofs. One particular area of interest is the construction of polyhedra, which involves understanding their underlying mathematical principles and theorems.
Construction of polyhedra involves creating three-dimensional shapes from flat polygons. This process requires a deep understanding of geometric principles, such as the properties of polygons and the concept of symmetry. By combining and manipulating these polygons, mathematicians are able to construct complex and intricate polyhedra.
One important concept in polyhedron construction is the idea of regular and convex polyhedra. Regular polyhedra are those in which all faces are congruent regular polygons, and the same number of polygons meet at each vertex. Convex polyhedra are those in which all points on a line segment connecting any two points within the shape lies inside the shape. These concepts provide a framework for constructing and classifying polyhedra based on certain criteria.
Mathematical theorems and proofs play a crucial role in exploring the construction of polyhedra. These theorems provide a rigorous foundation for the construction process, ensuring that the resulting polyhedra are mathematically valid. The use of proofs allows mathematicians to verify the correctness of various construction methods and techniques.
One well-known theorem in the field of polyhedron construction is Euler's formula, which relates the number of vertices, faces, and edges of a polyhedron. This formula, V - E + F = 2, provides valuable insights into the relationships between these different components and helps guide the construction process. Other theorems, such as those related to symmetry and tessellation, further enhance our understanding of polyhedron construction.
Visualizing the construction of polyhedra can be facilitated through the use of various mathematical tools and representations. One common tool is the use of nets, which are two-dimensional representations of the polyhedra that can be folded to create the three-dimensional shape. Nets provide a visual guide for understanding the relationships between different faces and vertices.
In addition to nets, the use of computer simulations and 3D modeling software has revolutionized the exploration of polyhedron construction. These tools allow mathematicians to visualize complex polyhedra and test different construction techniques in a virtual environment. Through these visual representations, mathematicians can gain further insights into the mathematical principles and relationships underlying the construction process.
• Polyhedron construction involves creating three-dimensional shapes from flat polygons.
• Regular and convex polyhedra provide a framework for constructing and classifying polyhedra.
• Mathematical theorems and proofs guide and validate the construction process.
• Visual tools such as nets, computer simulations, and 3D modeling enhance the understanding of polyhedron construction.
Polyhedra are three-dimensional objects with flat faces, straight edges, and sharp corners. They are a fundamental concept in geometry and have been studied for centuries. In order to better understand the properties and characteristics of different polyhedra, mathematicians have developed a classification system and naming convention.
The classification of polyhedra is based on the number of faces, edges, and vertices they have. There are several different types of polyhedra, including prisms, pyramids, and platonic solids. Each type has its own unique set of properties and symmetries.
Polyhedra are named using a combination of their type and the number of faces, edges, and vertices they have. For example, a polyhedron with six faces, eight vertices, and twelve edges is called a hexahedron. The names for polyhedra can be quite complex, but they provide a standardized way of referring to specific shapes.
Prism
Any
2n
n+2
Prism
Pyramid
n+1
3n
n+1
Pyramid
Platonic Solid
Any
Any
Any
Platonic Solid
By understanding the classification and naming of polyhedra, mathematicians and scientists can better communicate and analyze the properties of these complex geometric objects. The study of polyhedra has applications in a wide range of fields, including architecture, physics, and computer graphics.
In the field of mathematics, polyhedral networks are a fascinating subject of study. These networks consist of a collection of vertices, edges, and faces that are interconnected in a specific way. One fundamental concept in understanding polyhedral networks is the Euler formula, which relates the number of vertices, edges, and faces of a network.
The Euler formula states that for any polyhedral network that is topologically equivalent to a sphere, the following relationship holds: V - E + F = 2, where V represents the number of vertices, E represents the number of edges, and F represents the number of faces in the network.
This formula is named after the Swiss mathematician Leonhard Euler, who introduced it in the 18th century. It has since become a crucial tool in the study of polyhedral networks and has various applications in geometry and topology.
The proof of the Euler formula is based on the concept of planar graphs. A planar graph is a graph that can be drawn on a plane without any edges crossing. Polyhedral networks can be represented as planar graphs by mapping each face to a region in the plane and connecting the vertices and edges accordingly.
By considering such a planar representation of a polyhedral network, we can apply the following observations:
Each edge is shared by exactly two faces.
Each face is bounded by at least three edges.
Each vertex is connected to at least three edges.
Based on these observations, we can derive the following equations:
The total number of edges is equal to half the sum of the degrees of all vertices: E = (1/2) * ∑deg(v).
The total number of faces is equal to the sum of the degrees of all vertices minus twice the number of edges: F = ∑deg(v) - 2E.
Substituting these equations into the Euler formula V - E + F = 2, we get (1/2) * ∑deg(v) - V + ∑deg(v) - 2E = 2. Simplifying this expression, we obtain 2E - 2V + ∑deg(v) = 2, which can be further simplified as E - V + (1/2) * ∑deg(v) = 1.
Ultimately, this equation proves the Euler formula for polyhedral networks, stating that the difference between the number of edges and vertices plus half the sum of the degrees of all vertices is equal to 1 in a planar representation of a polyhedral network.
Regular and convex polyhedra have been the focus of mathematical study for centuries. These geometric figures possess a unique beauty and symmetry that has captivated mathematicians, architects, and artists alike. In this section, we will explore some of the key theories and concepts related to regular and convex polyhedra.
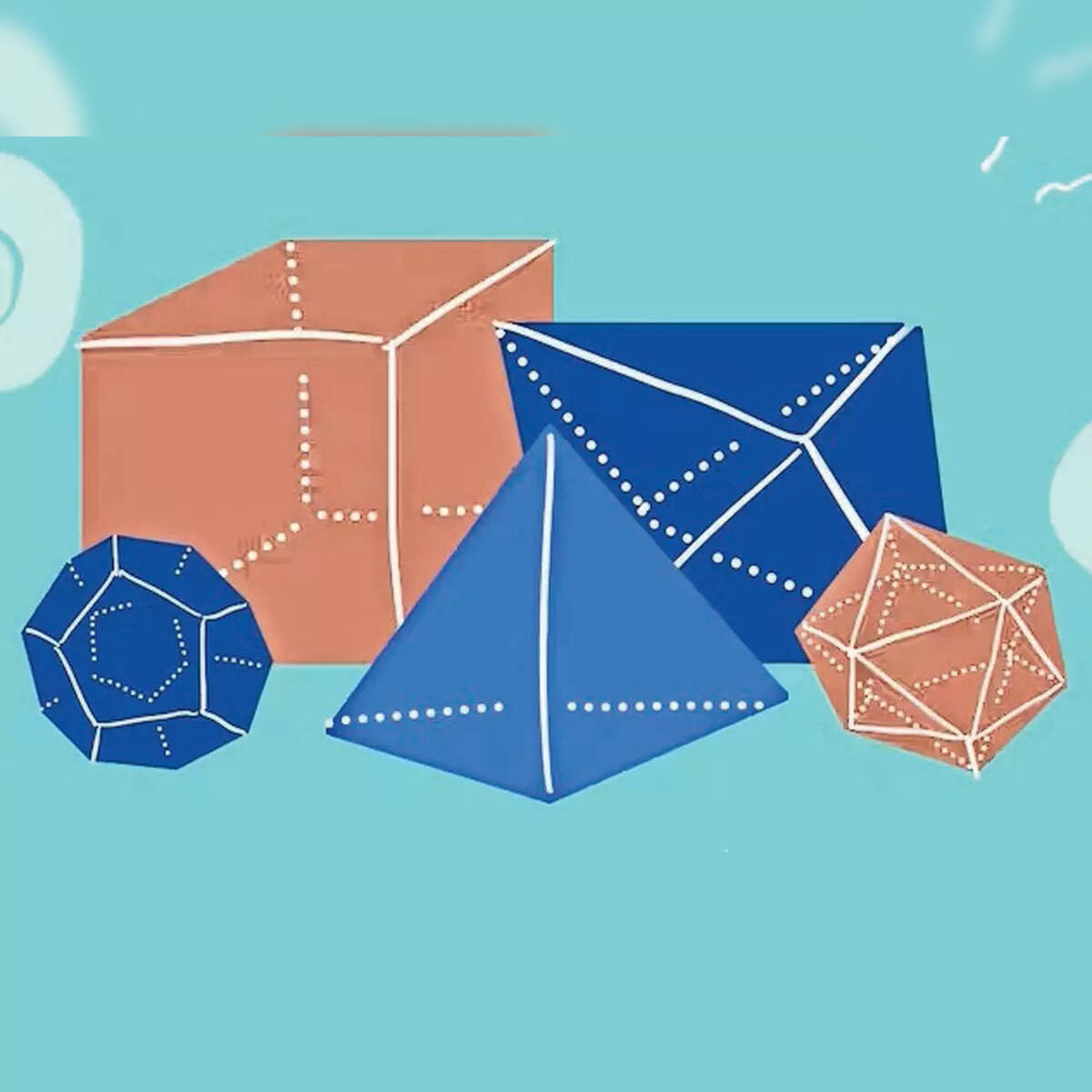
1. Regular Polyhedra: Regular polyhedra, also known as Platonic solids, are convex polyhedra whose faces are all congruent regular polygons. There are only five regular polyhedra: the tetrahedron, cube, octahedron, dodecahedron, and icosahedron. Each of these polyhedra has unique properties and characteristics, making them fascinating objects of study.
2. Euler's Formula: One of the fundamental theorems in the field of polyhedra is Euler's formula. It states that for any convex polyhedron, the number of vertices (V), edges (E), and faces (F) are related by the equation V - E + F = 2. This formula provides a deep insight into the structure and connectivity of polyhedral geometries.
3. Convex Polyhedra: Convex polyhedra are three-dimensional figures in which every line segment connecting two points within the figure lies entirely inside the figure. These polyhedra have flat faces and sharp edges, creating a distinct shape. Convex polyhedra have been extensively studied due to their applications in various fields of mathematics and science.
4. Archimedean Solids: Archimedean solids are a family of polyhedra that have regular polygonal faces and identical vertices, but not all faces are the same. These solids are a generalization of the regular polyhedra and offer a rich variety of symmetries and structures. There are 13 Archimedean solids, including the truncated icosahedron (the shape of a soccer ball) and the rhombicuboctahedron.
5. Johnson Solids: Johnson solids are another family of convex polyhedra that have regular polygonal faces, but the vertex figures are not necessarily identical. There are 92 Johnson solids, each with its own unique set of properties and symmetries. These solids have diverse shapes and forms, making them intriguing objects of mathematical investigation.
In conclusion, the theories and concepts surrounding regular and convex polyhedra provide a rich tapestry for exploration and investigation. From the elegance of regular polyhedra to the complexity of Archimedean and Johnson solids, these mathematical objects continue to fascinate and inspire researchers across various disciplines.
Solid geometry is a branch of mathematics that deals with the study of three-dimensional shapes, known as solids. One area of focus within solid geometry is the study of polyhedra, which are three-dimensional objects with flat polygonal faces, straight edges, and sharp corners or vertices.
The study of polyhedra involves the exploration of their properties, including the number of faces, edges, and vertices they possess. One important aspect of this study is the development of mathematical theories and proofs that not only describe these properties but also provide a deeper understanding of the nature of polyhedra.
Proofs play a crucial role in solid geometry as they provide the rigor and certainty necessary to establish the validity of mathematical statements. In the context of polyhedra, proofs are used to demonstrate the properties and characteristics of these three-dimensional objects.
Through the process of proof, mathematicians are able to show that certain relationships hold true for all polyhedra, regardless of their specific shapes or sizes. These relationships may involve the angles formed by the faces, the lengths of the edges, or the measures of the dihedral angles between adjacent faces.
By relying on theorems and logical reasoning, mathematicians are able to derive knowledge about polyhedra that goes beyond simple observation or intuition. The use of proofs enables the establishment of general principles and rules that can be applied to a wide variety of polyhedra, leading to a more comprehensive understanding of their properties.
Over the centuries, mathematicians have developed a wide range of proofs related to polyhedra. These proofs often involve the application of different mathematical techniques and concepts, including algebra, trigonometry, and coordinate geometry.
One of the fundamental concepts in the study of polyhedral proofs is Euler's formula, which relates the number of vertices, edges, and faces of a polyhedron. This formula, along with other theorems and propositions, serves as a starting point for the development of more intricate proofs in solid geometry.
By exploring the mathematical theories and proofs behind polyhedra, mathematicians continue to deepen our understanding of these fascinating three-dimensional objects. Their work not only contributes to the advancement of pure mathematics but also has practical applications in fields such as architecture, computer graphics, and crystallography.
Polyhedra, with their elegant geometric shapes and symmetries, have long been used as a source of inspiration in architecture and design. From ancient civilizations to modern-day constructions, polyhedra have played a significant role in shaping the built environment.
One of the key applications of polyhedra in architecture is in structural design. The geometry of polyhedra offers a highly efficient way to distribute forces and stresses, making them ideal for creating stable and durable structures. Architects and engineers often use polyhedral models to explore different structural possibilities and find the most optimal solution.
Polyhedra also find their place in architectural design, particularly in the creation of unique and eye-catching facades. By composing different polyhedral units, architects can achieve striking visual effects and play with light, shadow, and reflection. The intricate patterns formed by polyhedral structures can create a sense of movement and dynamism in buildings, adding a layer of complexity and interest.
The use of polyhedra is not limited to the exterior. Interior spaces can also benefit from the incorporation of polyhedral elements. By incorporating polyhedral shapes in ceilings, walls, and furniture, architects and designers can create a sense of spatial depth and complexity. Polyhedral geometries can also be used to define different zones within a space, guiding circulation and creating an immersive experience for users.
Polyhedra's aesthetic appeal and structural properties have also found application in industrial design. Furniture designers, for example, have utilized polyhedral forms to create unique and functional pieces. The angular shapes and precise proportions of polyhedra can lend themselves well to the creation of chairs, tables, and lighting fixtures that are both visually appealing and ergonomically sound.
Conclusion
In conclusion, the use of polyhedra in architecture and design offers a myriad of possibilities. From structural efficiency to visual aesthetics, polyhedra provide architects and designers with a versatile toolkit to create unique and innovative spaces. By incorporating polyhedral elements, architects and designers can push the boundaries of traditional design and create spaces that captivate and inspire.
What are galxe polyhedra?
Galxe polyhedra are a specific type of polyhedra that have a unique mathematical structure and properties. They are a fascinating subject of study in mathematics and have various applications in different fields.
Are there specific mathematical theories related to galxe polyhedra?
Yes, there are several mathematical theories and frameworks that have been developed to understand and analyze galxe polyhedra. These theories involve concepts such as graph theory, combinatorics, and group theory.
What are some of the key properties of galxe polyhedra?
Galxe polyhedra have several key properties that make them interesting. For example, they have a specific vertex arrangement, they can have symmetries and rotations, and they have certain mathematical formulas that describe their structure and characteristics.
What are some applications of galxe polyhedra?
Galxe polyhedra have applications in various fields, including computer graphics, crystallography, and materials science. They can be used to model and analyze the structure of molecules, design complex geometric shapes in computer graphics, and study the properties of crystalline materials.
Can you provide an example of a galxe polyhedron and its mathematical proof?
One example of a galxe polyhedron is the truncated icosahedron, which is also known as the soccer ball shape. It has 12 regular pentagons and 20 regular hexagons as its faces. The mathematical proof behind its structure involves analyzing the angles and edge lengths of its faces and vertices, as well as understanding its symmetries and rotational properties.
What are galxe polyhedra?
Galxe polyhedra are three-dimensional shapes formed by the combination of multiple regular polygons. They have a unique set of properties and are widely studied in mathematics.
What is the significance of the mathematical theories behind galxe polyhedra?
The mathematical theories behind galxe polyhedra help us understand the geometric properties and characteristics of these shapes. They provide a framework for analyzing and classifying different galxe polyhedra based on their symmetry, vertex configuration, and other mathematical properties.
How are the mathematical proofs behind galxe polyhedra constructed?
Mathematical proofs behind galxe polyhedra are constructed using logical reasoning and mathematical principles. They often involve applying various mathematical theorems, formulas, and techniques to establish the desired conclusions and properties of these shapes.
What are some practical applications of the mathematical theories and proofs behind galxe polyhedra?
The mathematical theories and proofs behind galxe polyhedra have practical applications in fields such as architecture, computer graphics, and physics. They help designers and engineers understand the structural properties of complex three-dimensional shapes and assist in designing efficient and aesthetically pleasing structures.
2022-2024 @ The mathematical theories and proofs behind galxe polyhedra
One useful tool for understanding polyhedra is Galxe's application modules. These modules allow users to interact with and manipulate different polyhedra, exploring their properties and visualizing their structure.