Galxe login | Galxe ID | Passport | Explore Campaigns | Galxe products
Galxe login | Galxe ID | Passport | Explore Campaigns | Galxe products
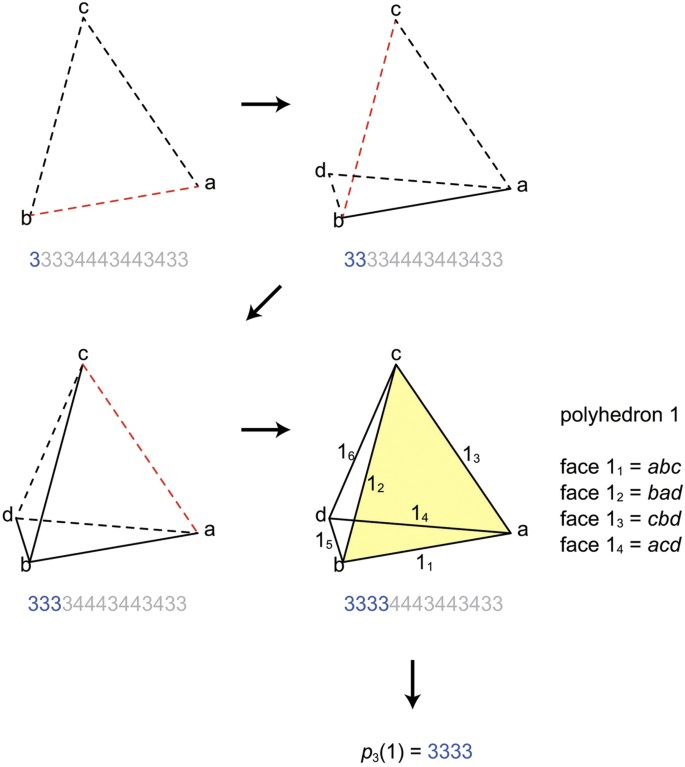
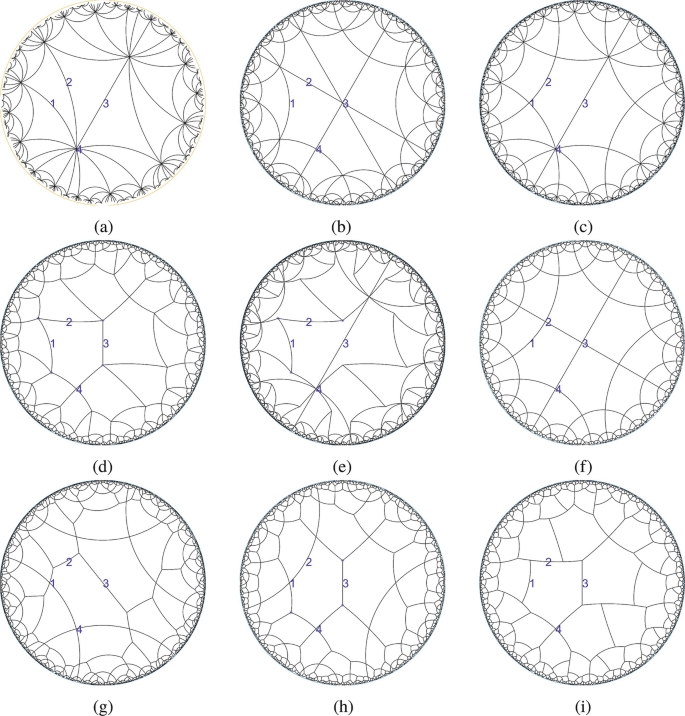
The exploration of symmetries and tilings has been a fascinating subject in mathematics for centuries. One intriguing aspect of this field is the study of galxe polyhedra – complex three-dimensional shapes with unique symmetries. These polyhedra are a source of wonder and inspiration for mathematicians, artists, and scientists alike.
Galxe polyhedra are characterized by their intricate patterns and symmetries, which can be analyzed and understood using various mathematical techniques. By studying the symmetries of galxe polyhedra, researchers can gain insights into the underlying structures and principles that govern their formation. This knowledge has applications in crystallography, architecture, computer graphics, and other fields.
The investigation of galxe polyhedra involves exploring different types of symmetries, such as rotational, reflectional, and translational symmetries. These symmetries give rise to beautiful patterns and repeatable motifs, which can be further classified into various tiling arrangements. By examining these tilings, mathematicians can uncover hidden relationships and connections between different galxe polyhedra.
Moreover, the study of galxe polyhedra extends beyond their symmetries and tilings. Researchers also investigate their geometrical properties, such as their surface area, volume, and convexity. These properties provide crucial information about the physical characteristics of galxe polyhedra and their potential applications in the real world.
In conclusion, the investigation of the symmetries and tilings of galxe polyhedra opens up a world of discovery and fascination. Through careful analysis and mathematical exploration, researchers can unravel the mysteries behind these intricate shapes and gain a deeper understanding of the underlying principles that govern their existence.
Galxe polyhedra are fascinating geometric structures that have captured the attention of mathematicians and researchers alike. These polyhedra, also known as Goldberg polyhedra, are named after Michael Goldberg, who first described them in the 1950s. They are constructed by taking a regular polyhedron and adding additional vertices within each face, resulting in a new, more intricate polyhedral shape.
The study of galxe polyhedra is a complex and important field within geometry and topology. These structures exhibit symmetrical patterns and tilings, providing insight into the underlying symmetries of regular polyhedra. By exploring the properties of galxe polyhedra, mathematicians can gain a deeper understanding of fundamental mathematical concepts and discover new connections between different areas of mathematics.
One way to visualize and analyze galxe polyhedra is through the Galxe project. The Galxe project is a comprehensive initiative that aims to provide an overview, analytics, and data related to galxe polyhedra. By visiting the Galxe - Project Overview, Analytics, and Data, researchers can access a wealth of information about galxe polyhedra, including their classification, symmetries, and tiling properties.
The symmetries and tilings of galxe polyhedra are fundamental elements that contribute to their unique and captivating properties. Symmetry, in particular, plays a crucial role in understanding the structure and behavior of these polyhedra. By examining the symmetries present in a galxe polyhedron, mathematicians can identify patterns, relationships, and other mathematical properties.
Tilings, on the other hand, involve covering a plane or space with congruent copies of a particular shape. The tilings of galxe polyhedra can be intricate and intricate, leading to interesting visual patterns and mathematical structures. Exploring the various tilings of galxe polyhedra is an essential aspect of understanding their overall geometry and topology.
Overall, the study of galxe polyhedra and their symmetries and tilings is a captivating field that offers valuable insights into the world of mathematics. By delving into the intricacies of these geometric structures and utilizing tools like the Galxe project, mathematicians can continue to expand our knowledge of symmetries, tilings, and their connections to other mathematical concepts.
Symmetry plays a crucial role in the study of galxe polyhedra. These polyhedra are three-dimensional structures composed of interconnected polygons, and their symmetries provide valuable insights into their properties and characteristics.
One of the main reasons why symmetries are significant in galxe polyhedra is that they can help determine the overall structure and arrangement of the polygons. By analyzing the symmetries, researchers can identify the repeating patterns and motifs within the polyhedra, which can provide useful information about their overall geometry.
Moreover, symmetries also have implications for the physical properties of galxe polyhedra. The symmetrical arrangement of polygons can affect various properties such as the stability, rigidity, and strength of the structure. Understanding these symmetries allows researchers to gain a deeper understanding of how these properties emerge and how they can be manipulated for different purposes.
Additionally, symmetries in galxe polyhedra can have aesthetic and artistic implications. The recurring patterns and symmetrical arrangements within these structures often give them a visually appealing and harmonious quality. By studying and exploiting these symmetries, researchers and designers can create visually striking and aesthetically pleasing structures.
To aid in the analysis and understanding of symmetries, researchers often use tables to describe the different symmetries present in galxe polyhedra. These tables typically list the types of symmetries, such as rotational symmetries or mirror symmetries, along with their corresponding features and transformations.
Rotational symmetry
Rotation around a point
90°, 180°, 270°
Mirror symmetry
Reflection across a line
Horizontal, vertical
Translational symmetry
Shift along a direction
Up, down, left, right
By studying these symmetries and their corresponding transformations, researchers can gain a comprehensive understanding of the symmetrical properties of galxe polyhedra and their significance in various fields such as mathematics, physics, and design.
Galxe polyhedra are fascinating geometric structures that exhibit intricate symmetries and tilings. These polyhedra are formed by connecting regular polygons together in a way that fills three-dimensional space without gaps or overlaps. Their symmetrical arrangements and mesmerizing patterns have captivated mathematicians and scientists for centuries.
One of the most remarkable features of galxe polyhedra is their symmetries. These symmetries can be classified into different types, such as rotational symmetries, reflection symmetries, and glide reflections. Each type of symmetry creates unique patterns and arrangements of the polygons, leading to an array of beautiful and visually stunning structures.
Exploring the symmetries of galxe polyhedra allows us to uncover hidden patterns and relationships within these intricate structures. By analyzing the symmetries, we can determine the number of distinct polyhedral forms, understand the underlying mathematical principles, and even predict new types of polyhedra that have yet to be discovered.
Tilings play a crucial role in studying and understanding galxe polyhedra. A tiling is a covering of a plane or a three-dimensional space using one or more shapes, without any gaps or overlaps. By examining the tilings of galxe polyhedra, mathematicians can gain insights into the underlying symmetries and spatial arrangements of the polygons.
The study of tilings in galxe polyhedra involves exploring different types of tilings, such as regular tilings, semi-regular tilings, and non-regular tilings. Each type of tiling reveals a different aspect of the polyhedra's structure and symmetries, allowing us to delve deeper into the hidden beauty of these geometric wonders.
The study of galxe polyhedra has numerous applications in various fields, including crystallography, material science, and architecture. Understanding the symmetries and tilings of these structures can aid in the design and development of new materials with specific properties.
Furthermore, the exploration of galxe polyhedra continues to be an active area of research, with researchers constantly discovering new types of polyhedra and uncovering new symmetries and tilings. As we delve deeper into the hidden beauty of galxe polyhedra, we gain a deeper understanding of the fundamental principles that govern the world of geometry and mathematics.
In conclusion, galxe polyhedra are not just fascinating geometric structures; they are windows into a world of hidden beauty. By investigating their symmetries and tilings, we unlock the secrets of these intricate structures and gain a deeper appreciation for the elegance and complexity of mathematics.
Galxe polyhedra are fascinating geometric structures that exhibit a variety of symmetries and tilings. By examining the patterns of these tilings, we can gain a deeper understanding of the complex relationships between the faces, vertices, and edges of these polyhedra.
One of the ways to investigate the tilings of galxe polyhedra is to analyze the recurring patterns that appear on the faces. These patterns can be simple or more intricate, depending on the specific polyhedron. By identifying the underlying rules governing these patterns, we can begin to uncover the symmetries and symmetrical transformations that give rise to the tiling arrangements.
Some galxe polyhedra exhibit regular tilings, where identical tiles are repeated in a systematic fashion to cover the entire surface. Others may have irregular or aperiodic tilings, where the arrangement of tiles does not repeat in a predictable manner. Understanding the properties of these tilings can provide valuable insights into the geometric properties of the polyhedra.
The study of symmetries in galxe polyhedra involves identifying the various symmetry operations that can be performed on the polyhedra while preserving their overall shape and structure. These operations can include rotations, reflections, translations, and combinations of these transformations.
By examining the transformations that preserve the tiling patterns, we can determine the symmetries that are present in the polyhedra. For example, a polyhedron may exhibit rotational or reflectional symmetry, meaning that it can be rotated or reflected to match its original configuration. Additionally, translational symmetries can arise when the polyhedron can be shifted by a certain distance and still maintain its overall shape.
The investigation of the tilings and symmetries of galxe polyhedra has several practical applications in various fields. In mathematics, the study of these structures can provide insights into the fundamental principles of geometry and topology. Additionally, the symmetries and tilings of galxe polyhedra can be utilized in architectural design, crystallography, and even the development of new materials with specific geometric properties.
Overall, exploring the tilings and symmetries of galxe polyhedra is a fascinating endeavor that allows us to delve into the intricate patterns and geometric properties of these unique structures. By unraveling the mysteries of these polyhedra, we can uncover new knowledge and applications across various disciplines.
Galxe polyhedra are a fascinating class of geometric shapes that exhibit mesmerizing patterns and symmetries. These polyhedra have captured the attention of mathematicians, artists, and scientists alike due to their intricate designs and aesthetic appeal.
One of the most striking features of galxe polyhedra is their ability to tile three-dimensional space. Tiling refers to the process of covering a plane or space with geometric shapes without any gaps or overlaps. Galxe polyhedra possess unique properties that enable them to fill space in a variety of ways, creating captivating patterns and structures.
These polyhedra are characterized by their symmetrical arrangements of faces, edges, and vertices. Symmetry plays a crucial role in the formation of these mesmerizing patterns. The symmetries of galxe polyhedra can be classified into different types, such as rotational, reflectional, and glide symmetries. These symmetries give rise to repetitive motifs and mirror-like reflections, adding to the aesthetic appeal of the polyhedra.
Through the study of galxe polyhedra, mathematicians have discovered numerous interesting properties and relationships. For example, certain polyhedra can be transformed into each other through specific operations, such as rotations, translations, or scaling. These transformations reveal hidden symmetries and connections between different polyhedra, further enhancing our understanding of their mesmerizing patterns.
Galxe polyhedra have also found applications in various fields, including architecture, design, and materials science. The unique geometries and symmetries of these polyhedra provide inspiration for the creation of innovative structures and materials. Researchers are exploring the use of galxe polyhedra in the development of new building materials, advanced manufacturing techniques, and even artistic installations.
In conclusion, the mesmerizing patterns of galxe polyhedra have fascinated and captivated individuals from diverse disciplines. The intricate designs, symmetrical arrangements, and tiling properties of these polyhedra make them a subject of great interest and exploration. As scientists continue to investigate these mesmerizing patterns, we can expect to uncover even more fascinating insights and applications.
The study of galxe polyhedra and their symmetries and tilings has diverse applications in various fields. These unique structures have captured the interest of researchers, scientists, and mathematicians. Here are some of the applications where galxe polyhedra are being utilized.
1. Material Science: The symmetries and properties of galxe polyhedra can be used in designing new materials with unique characteristics. By understanding the symmetries of these structures, scientists can create materials with desired properties, such as enhanced strength or increased conductivity.
2. Architecture and Design: The tilings of galxe polyhedra can inspire new architectural designs. Architects can use these structures to create aesthetically pleasing patterns and forms that are visually interesting and structurally sound.
3. Computer Graphics and Animation: Galxe polyhedra can be used in computer graphics and animation to create complex and visually appealing models. Their symmetries and tilings can be utilized to generate intricate patterns and designs, adding a realistic touch to virtual environments.
4. Mathematics and Geometry: Galxe polyhedra provide valuable insights into the mathematical properties of three-dimensional space. They can be used to study symmetry groups, tessellations, and topological properties, contributing to the advancement of mathematical theory.
5. Nanotechnology: The unique properties of galxe polyhedra make them potential building blocks for nanoscale devices. Researchers are exploring ways to use these structures in the construction of nanoscale circuits, sensors, and other miniaturized devices.
6. Art and Sculpture: The intricate patterns and symmetrical designs of galxe polyhedra have also found their way into art and sculpture. Artists and sculptors can draw inspiration from these structures to create visually stunning and mathematically precise artworks.
Overall, the study of galxe polyhedra has far-reaching applications across various fields, ranging from materials science to computer graphics, mathematics, nanotechnology, and art. The unique symmetries and tilings of these polyhedra open up new avenues for exploration and innovation.
What are galxe polyhedra?
Galxe polyhedra are a class of three-dimensional geometric structures that can be obtained by projecting certain thinned polyhedra onto a sphere.
What is the significance of investigating symmetries and tilings of galxe polyhedra?
Investigating the symmetries and tilings of galxe polyhedra helps in understanding the underlying mathematical principles and patterns that govern their formation. It also has potential applications in fields such as crystallography, architecture, and physics.
How can galxe polyhedra be used in crystallography?
Galxe polyhedra can provide insights into the atomic arrangement and symmetry properties of crystals. By studying their symmetries and tilings, scientists can gain a better understanding of the structures and properties of various crystal systems.
Are galxe polyhedra limited to specific shapes?
No, galxe polyhedra can be derived from a wide range of polyhedra, including regular and irregular ones. The formation of galxe polyhedra depends on the specific thinned polyhedron used as the base shape.
Can the concept of galxe polyhedra be extended to higher dimensions?
While the concept of galxe polyhedra primarily applies to three-dimensional space, similar principles can be extended to higher dimensions. The investigation of higher-dimensional galxe polytopes and their symmetries is an active area of mathematical research.
What are galxe polyhedra?
Galxe polyhedra are a type of geometric shape that are composed of multiple polygons. They are named after their creator, mathematician Galileo Galilei.
What are the symmetries of galxe polyhedra?
The symmetries of galxe polyhedra depend on the number and arrangement of polygons that make up the shape. Some have rotational symmetry, while others have reflectional symmetry.
How are galxe polyhedra different from regular polyhedra?
Galxe polyhedra are different from regular polyhedra in that their faces are not all the same shape or size. Regular polyhedra have identical faces, while galxe polyhedra can have a combination of different polygons.
Why are galxe polyhedra important in mathematics?
Galxe polyhedra are important in mathematics because they provide a way to study the relationship between shapes and symmetries. They can also be used to create interesting visual patterns and designs.
Galxe login | Galxe ID | Passport | Explore Campaigns | Galxe products
2022-2024 @ Investigating the symmetries and tilings of galxe polyhedra
